
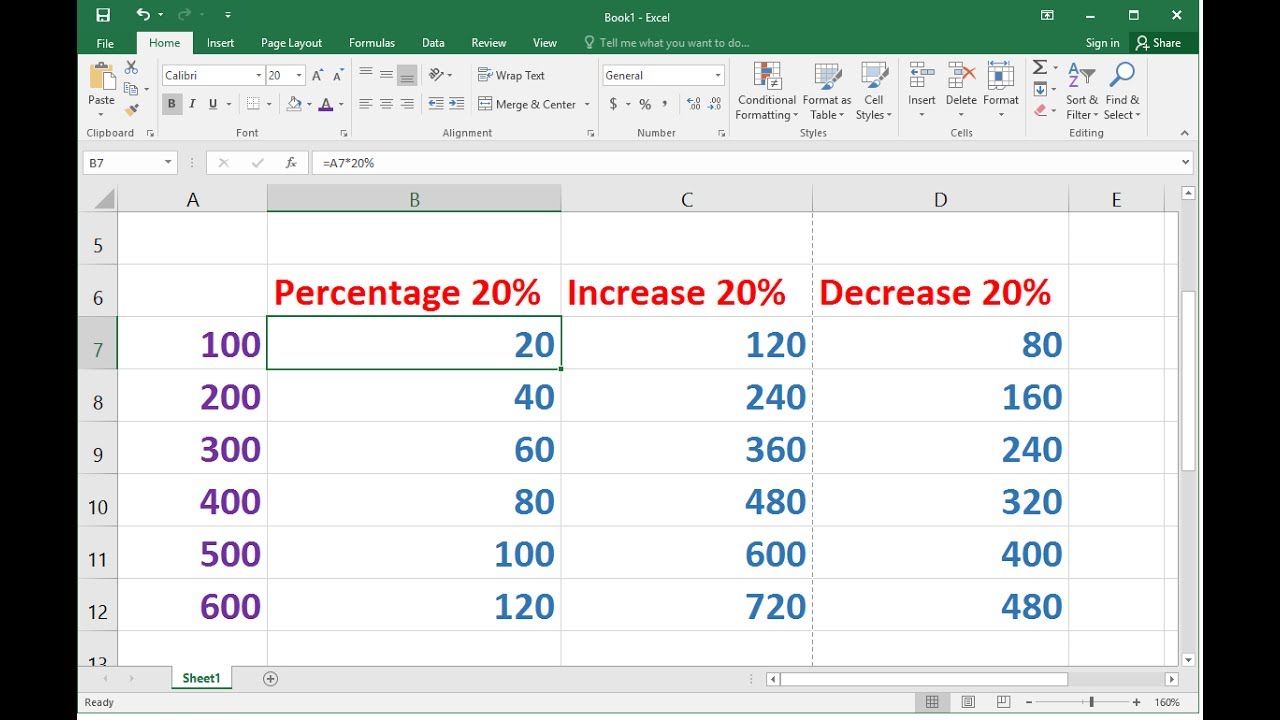
This can also be thought of as 6/10 of 100, which is of course 60.Īs in any mathematics, estimating skills are important. Using a formula to calculate a percentage requires an understanding of what is happening: the numerator of a fraction is divided by the denominator giving a decimal fraction which is then multiplied by 100 to express a percentage. 15% = 10% + half of 10%) and simplifying fractions and expressing these as percentages (eg. Students will come to recognise the advantages of working with decimals rather than fractions when converting to percentages, particularly when the ratio cannot be represented as a common fraction.īuilding a ready knowledge of common equivalent fractions for percentages, (1/4 = 25%, 1/2 = 50%, 3/4 = 75%, 1/10 = 10%, 1/5 = 20%, 1/8 = 12.5%), being able to use these as benchmarks to work out some other percentages (eg. With this understanding a student can readily see, for example, that 0.6 is 6 tenths, is also 60 hundredths and is therefore 60%, whilst 0.06 is 6 hundredths and is therefore just 6%. Having a sound decimal place value understanding underpins the students’ ability to work with percentages. 75/100 (or 3/4), percentage 75% and decimal representation 0.75 of the same amount. It is important to use a range of physical representations to ensure that the students can clearly see and make the connections between the fraction: eg. Therefore, a percentage can be thought of as a way of expressing a fraction of 100, another way of writing hundredths, and a new way of expressing the concept ‘out of 100’, using the % notation.

The symbol % is made up of the / per sign and the two zeros (00) from the number 100. Percent, from the Latin per centum literally means out of (per) one hundred (cent). Relational thinking underpins the students' ability to understand that a percentage is used to express how large or small one quantity is in relation to another quantity (which is greater than zero). It is important that students are given opportunities to explore, recognise, demonstrate and articulate these connections for themselves, and to be able to work fluently between them. The ability to understand and work competently with percentages depends on the students having a sound understanding of place value, of our decimal number system and of fractions and their operations.


 0 kommentar(er)
0 kommentar(er)
